Team:ETH Zurich/labblog/20140824mod
From 2014.igem.org
(→Monday, August 24th) |
(→Fitting quorum sensing dynamic curves) |
||
| Line 13: | Line 13: | ||
$$ \frac{d[GFP]}{dt}=\frac{k_{45}[P_{tot}][AHLi]^2e^{-2d_{AHL}t}}{K_{d4}K_{d3}}-d_{GFP}[GFP]$$ | $$ \frac{d[GFP]}{dt}=\frac{k_{45}[P_{tot}][AHLi]^2e^{-2d_{AHL}t}}{K_{d4}K_{d3}}-d_{GFP}[GFP]$$ | ||
| - | We find $$[GFP]=\frac{k_{45}[P_{tot}][AHLi]^2}{K_{d4}K_{d3}(d_{GFP}-2d_{AHL})}(e^{-2d_{AHL}t}-e^{-d_{GFP}t})$$. | + | We find |
| + | $$ [GFP]=\frac{k_{45}[P_{tot}][AHLi]^2}{K_{d4}K_{d3}(d_{GFP}-2d_{AHL})}(e^{-2d_{AHL}t}-e^{-d_{GFP}t})$$ . | ||
This curve has a maximum at | This curve has a maximum at | ||
| - | $$t_{max}=\frac{1}{d_{GFP}-2d_{AHL}}ln\big(\frac{d_{GFP}}{2d_{AHL}}\big)$$ | + | $$ t_{max}=\frac{1}{d_{GFP}-2d_{AHL}}ln\big(\frac{d_{GFP}}{2d_{AHL}}\big)$$ |
This way we can find from experimental curves $$d_{AHL}=4,0.10^{-3} min^{-1}$$ | This way we can find from experimental curves $$d_{AHL}=4,0.10^{-3} min^{-1}$$ | ||
| - | This AHL degradation rate is alumped parameter between internal and external degradation rates, equivalent to $$d_{AHLext}+\alpha d_{AHLint}$$ where | + | This AHL degradation rate is alumped parameter between internal and external degradation rates, equivalent to $$d_{AHLext}+\alpha d_{AHLint}$$ where &alpha is the fraction of the volume occupied by cells in the whole culture. From that on we could find reasonable values for internal and external AHL degradation rates. |
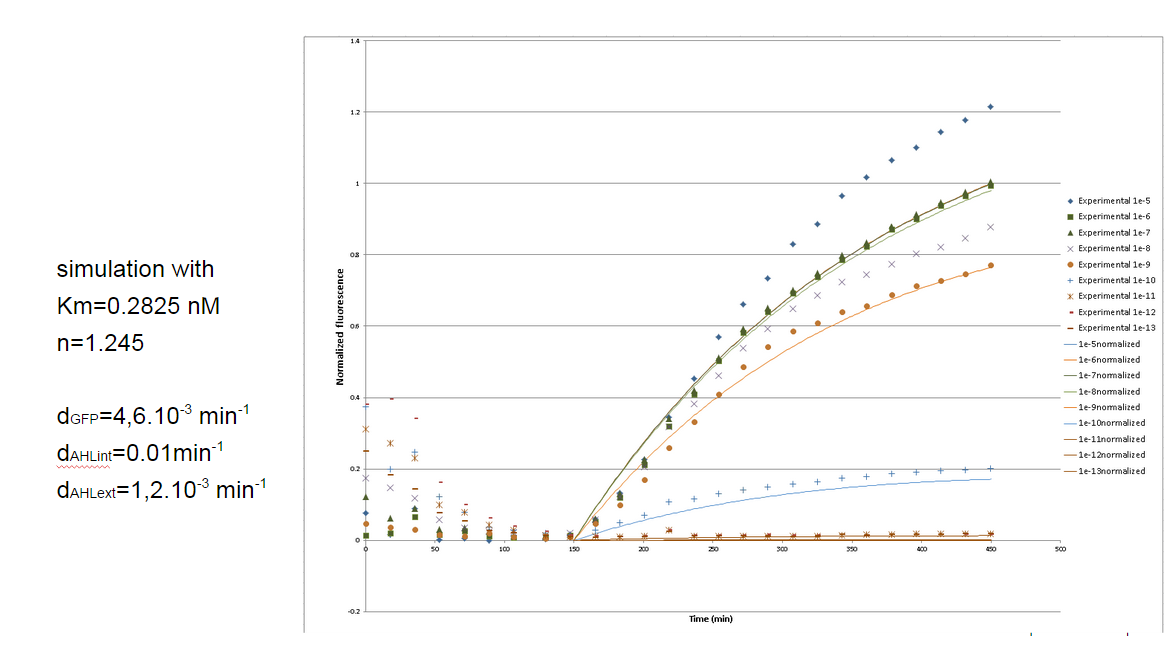
Finally the curves simulated by our model with these parameters fit the experiments quite well: | Finally the curves simulated by our model with these parameters fit the experiments quite well: | ||
Revision as of 19:57, 11 October 2014
Fitting quorum sensing dynamic curves
Monday, August 24th
We could use quorum sensing dynamic curves to fit degradation rates of external, internal AHL and GFP. We fitted the experimental results for lux with riboregulator and thus didn't consider leakiness (we showed leakiness was low enough in that case when fitting quorum sensing Hill function parameters ). By writing GFP production rate and replacing AHL in this equation by $$[AHL]=[AHL_i]-[AHL_{bound}]$$ we find $$\frac{d[GFP]}{dt}=\frac{k_{45}[P_{tot}]\Big(-1+\sqrt{1+8\frac{AHL_i}{K_{d3}}}\Big)^2}{16\frac{K_{d4}}{K_{d3}}+\Big(-1+\sqrt{1+8\frac{AHL_i}{K_{d3}}}\Big)^2}-d_{GFP}[GFP]$$. For very high initial concentrations AHLi, we have $$\frac{d[GFP]}{dt}=k_{45}[P_{tot}]-d_{GFP}[GFP]$$
so by taking $$t_{1/2}=\frac{ln(2)}{d_{GFP}}$$ from experimental curves, we are able to retrieve $$d_{GFP} = 4.9 . 10^{-3} min^{-1}$$.
For very low initial concentrations of initial AHL and considering degradation, we have
$$ \frac{d[GFP]}{dt}=\frac{k_{45}[P_{tot}][AHLi]^2e^{-2d_{AHL}t}}{K_{d4}K_{d3}}-d_{GFP}[GFP]$$
We find
$$ [GFP]=\frac{k_{45}[P_{tot}][AHLi]^2}{K_{d4}K_{d3}(d_{GFP}-2d_{AHL})}(e^{-2d_{AHL}t}-e^{-d_{GFP}t})$$ .
This curve has a maximum at
$$ t_{max}=\frac{1}{d_{GFP}-2d_{AHL}}ln\big(\frac{d_{GFP}}{2d_{AHL}}\big)$$
This way we can find from experimental curves $$d_{AHL}=4,0.10^{-3} min^{-1}$$
This AHL degradation rate is alumped parameter between internal and external degradation rates, equivalent to $$d_{AHLext}+\alpha d_{AHLint}$$ where &alpha is the fraction of the volume occupied by cells in the whole culture. From that on we could find reasonable values for internal and external AHL degradation rates.
Finally the curves simulated by our model with these parameters fit the experiments quite well:
 "
"