Team:Imperial/Mechanical Testing
From 2014.igem.org
(Created page with "{{:Team:Imperial/Templates:header}} <html> <body> <div class="content-wrapper"> <div class="pure-g"> <div class="pure-u-1-1 main"> ...") |
|||
| Line 56: | Line 56: | ||
<figure class="content-image"> | <figure class="content-image"> | ||
| - | <img class="image-full" src="https://static.igem.org/mediawiki/2014/6/60/IC14-MechTest-CelluloseTest.jpg"> | + | <img class="image-full image-half" src="https://static.igem.org/mediawiki/2014/6/60/IC14-MechTest-CelluloseTest.jpg"> |
</figure> | </figure> | ||
Revision as of 19:25, 15 October 2014
Mechanical Testing
Overview
Zombie ipsum reversus ab viral inferno, nam rick grimes malum cerebro. De carne lumbering animata corpora quaeritis. Summus brains sit, morbo vel maleficia? De apocalypsi gorger omero undead survivor dictum mauris. Hi mindless mortuis soulless creaturas, imo evil stalking monstra adventus resi dentevil vultus comedat cerebella viventium. Qui animated corpse, cricket bat max brucks terribilem incessu zomby. The voodoo sacerdos flesh eater, suscitat mortuos comedere carnem virus. Zonbi tattered for solum oculi eorum defunctis go lum cerebro. Nescio brains an Undead zombies. Sicut malus putrid voodoo horror. Nigh tofth eliv ingdead.
Key Achievements
Introduction



Perhaps the simplest assessment of the quality of any material is ‘how strong might it be’, which can be tested by pulling a test piece until it breaks, a tensile test. The quality of our bacterial cellulose will determine the water pressure that can be applied to it in a membrane bioreactor setting as the filter will be used in a flat orientation. Since the water pressure is the major limiting factors of the flow rates produced in such a setting, a key step to establishing the feasibility of our project is to perform a tensile test.
Tensile testing of bacterial cellulose is a common way of characterising and comparing its mechanical properties (Sherif 2006, Cheng 2009, Shezad 2010) and provides an indication of the orientation of the fibres in the bacterial cellulose pellicle. Therefore, we contacted Dr. Angelo Karunaratne of the Royal British Legion Centre of Blast Injury Studies at Imperial College, requesting access to test samples in an Instron 5866 materials testing machine (Instron Inc., Norwood, USA). Luckily Angelo was welcoming to the idea and allowed us to perform tensile stress-strain tests of our material. The aim was to calculate the young’s modulus, maximum stress applied and strain at failure.
Methods

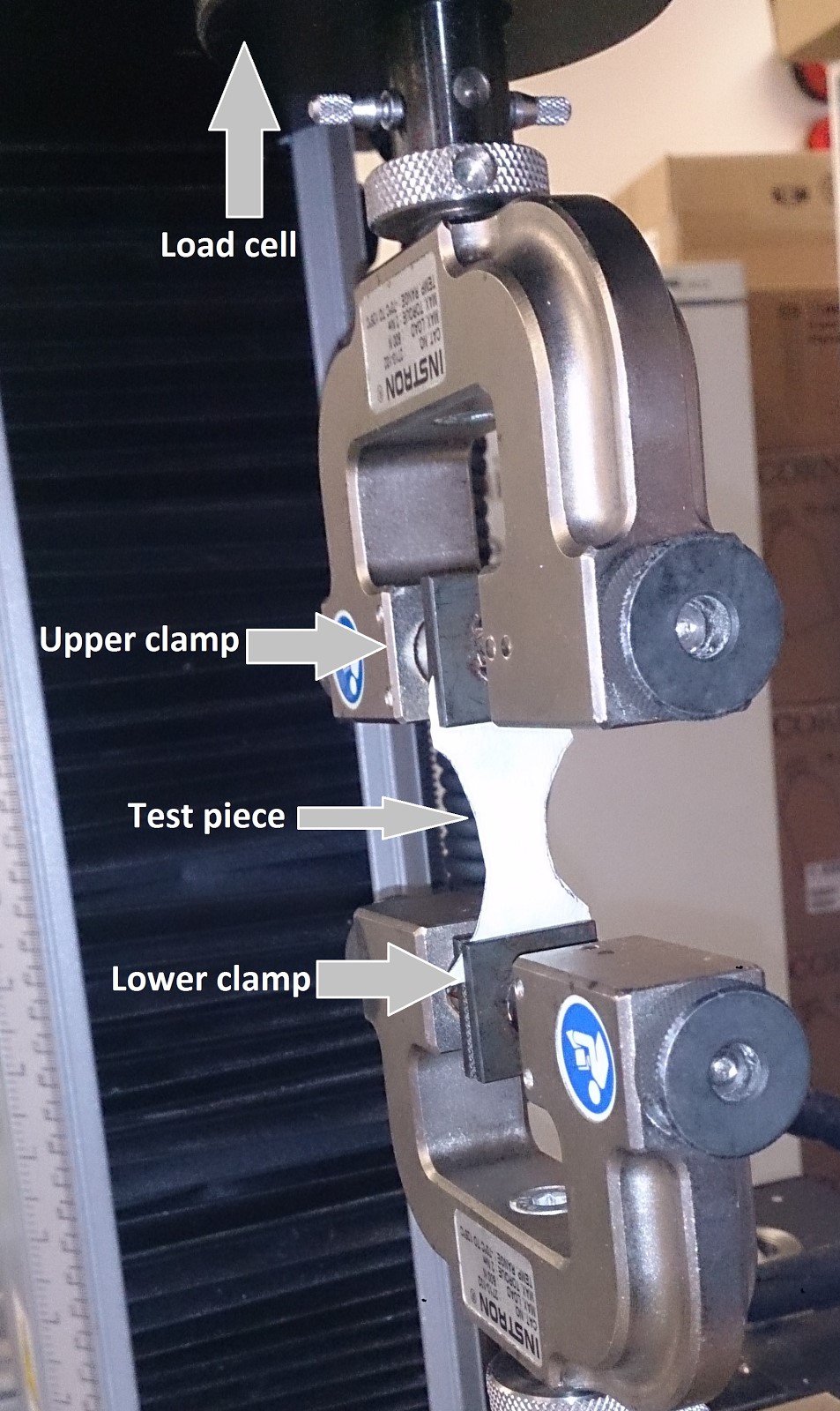

Samples were prepared by oven drying at 60°C for 6 hours between VWR 413 filter paper, without pressure. Subsequently, samples were kept at room temperature (20°C) before testing. Cellulose was cut into 0.1 mm x 11 mm x 32 mm dumbbell shapes according to the recommendation from the ISO 527-3 guidelines for determination of tensile properties of plastics. Dumbbell shapes have the advantage of avoiding stress concentration at the clamps, and the dimensions chosen were sufficiently similar to existing literature dimensions, 0.16 mm×100 mm×150 mm to allow for comparison across studies (Sherif 2006). A digital vernier calliper was used to measure the thickness of each of the samples. 8 samples were used for the main cellulose fabric intended for water filtration, fewer were used for alternatively processed samples due to limited availability.
The test involves clamping test pieces into the Instron machine, moving the upper clamp upwards at a specific rate, ‘elongation time’, set to 20 mm/min to match that of existing literature (Sherif, 2006) and tracking the tensile force this introduces in the BC sample until the sample breaks, failure. Stress(σ) was calculated as the tensile force divided by the cross sectional area measured as width x thickness. Young’s modulus was calculated as stress/strain. Strain (ε) was calculated as ΔL/L0where ΔL is the elongation from the initial length L0.
 "
"











